SAT & ACT Math: One Problem, Three Ways

On standardized college admissions tests, déjà vu is a good thing.
When tutoring for the SAT or ACT math sections, I like to tell students that both exams take basic problems and dress them up just enough to make them seem unfamiliar. Whether through the specific language they use or the way they manipulate expressions or figures, the test writers are expert at convincing students that the math they're seeing is beyond their comprehension. This helps the testing organizations achieve a nice bell curve distribution of scores, but it can make the student testing experience frustrating.
For example, consider this group of problems.
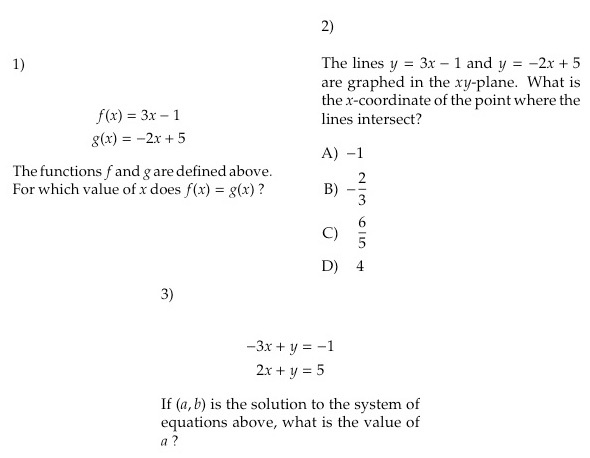
To the untrained eye, these look like three distinct questions. We see function notation in the first; we have a reference to coordinate geometry ("the xy-plane") in the second; we learn about a "system of equations" in the third. One question is multiple choice, while the others require a student-produced response ("grid-in").
For all practical purposes, though, these three questions are really the same problem, asking for the same thing, yielding the same solution (6/5, if you're interested). Easy, right? Not so fast.
To a student under the pressure of time constraints, these questions can seem completely different from one another. In order to understand why, we must consider how students learn math. Problem (2) above is a question students might encounter in coordinate geometry. Depending on their curriculum, this might have been addressed as early as 7th or 8th grade...or perhaps not until 9th, if the school teaches an integrated geometry curriculum. Later, students might cover "systems of equations" as a separate topic. Function notation might not come in until Algebra I. Notably, in some math curricula, there is overlap between problem types, and students will have seen the full span of problems under a given category. Still, many students will learn these as distinct topics, and thus might never synthesize the unifying concepts that underlie all of them. In short, the disjointed nature of much of our math education can make it difficult for students to connect the dots. (This, by the way, isn't to blame teachers, who generally do the best job they can do with the curricula they're given to teach. We need to overhaul the way we teach math, but that's a topic for a different post.)
This is why preparing for the SAT and ACT is so important. Learning how seemingly different problems are really asking for the same thing can enable students to take advantage of the tests' predictability. Without a focus on broad strategies that apply across topics and join apparently disparate concepts, students can find themselves at a great disadvantage. The good news is that expert tutors, well-crafted programs, and focused classes can help students of all stripes see the connections between problems and vastly improve their scores.


